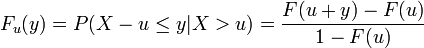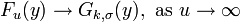Pickands–Balkema–de Haan theorem
The Pickands–Balkema–de Haan theorem is often called the second theorem in extreme value theory. It gives the asymptotic tail distribution of a random variable X, when the true distribution F of X is unknown. Unlike for the first theorem (the Fisher–Tippett–Gnedenko theorem) in extreme value theory, the interest here is in the values above a threshold.
Conditional excess distribution function
If we consider an unknown distribution function 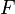 of a random variable
of a random variable 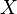 , we are interested in estimating the conditional distribution function
, we are interested in estimating the conditional distribution function 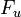 of the variable
of the variable 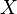 above a certain threshold
above a certain threshold 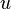 . This is the so-called conditional excess distribution function, defined as
. This is the so-called conditional excess distribution function, defined as
for 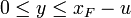 , where
, where 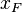 is either the finite or infinite right endpoint of the underlying distribution
is either the finite or infinite right endpoint of the underlying distribution 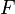 . The function
. The function 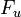 describes the distribution of the excess value over a threshold
describes the distribution of the excess value over a threshold 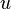 , given that the threshold is exceeded.
, given that the threshold is exceeded.
Statement
Let 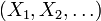 be a sequence of independent and identically-distributed random variables, and let
be a sequence of independent and identically-distributed random variables, and let 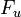 be their conditional excess distribution function. Pickands (1975), Balkema and de Haan (1974) posed that for a large class of underlying distribution functions
be their conditional excess distribution function. Pickands (1975), Balkema and de Haan (1974) posed that for a large class of underlying distribution functions 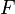 , and large
, and large 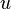 ,
, 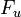 is well approximated by the generalized Pareto distribution. That is:
is well approximated by the generalized Pareto distribution. That is:
where
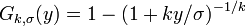 , if
, if 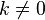
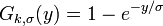 , if
, if 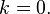
Here σ > 0, and y ≥ 0 when k ≥ 0 and 0 ≤ y ≤ −σ/k when k < 0. Since a special case of the generalized Pareto distribution is a power-law, the Pickands–Balkema–de Haan theorem is sometimes used to justify the use of a power-law for modeling extreme events. Still, many important distributions, such as the normal and log-normal distributions, do not have extreme-value tails that are asymptotically power-law.
Special cases of generalized Pareto distribution
- Exponential distribution with mean
 , if k = 0.
, if k = 0. - Uniform distribution on
![[0,\sigma]](../I/m/db6444586dbeaae8fefd71d9824de212.png) , if k = -1.
, if k = -1. - Pareto distribution, if k < 0.
Related subjects
References
- Balkema, A., and de Haan, L. (1974). "Residual life time at great age", Annals of Probability, 2, 792–804.
- Pickands, J. (1975). "Statistical inference using extreme order statistics", Annals of Statistics, 3, 119–131.