Pairwise Stone space

In mathematics and particularly in topology, pairwise Stone space is a bitopological space 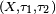\scriptstyle (X,\tau_1,\tau_2) which is pairwise compact, pairwise Hausdorff, and pairwise zero-dimensional.

Pairwise Stone spaces are a bitopological version of the Stone spaces.

Pairwise Stone spaces are closely related to spectral spaces.

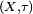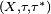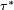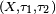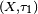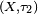Theorem:[1] If \scriptstyle (X,\tau) is a spectral space, then \scriptstyle (X,\tau,\tau^*) is a pairwise Stone space, where \scriptstyle \tau^* is the de Groot dual topology of \scriptstyle \tau . Conversely, if \scriptstyle (X,\tau_1,\tau_2) is a pairwise Stone space, then both \scriptstyle (X,\tau_1) and \scriptstyle (X,\tau_2) are spectral spaces.

See also

Notes

  1. ↑ G. Bezhanishvili, N. Bezhanishvili, D. Gabelaia, A. Kurz, (2010). Bitopological duality for distributive lattices and Heyting algebras. Mathematical Structures in Computer Science, 20.
This article is issued from Wikipedia - version of the 5/27/2013. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.