Method of images
The method of images (or method of mirror images) is a mathematical tool for solving differential equations, in which the domain of the sought function is extended by the addition of its mirror image with respect to a symmetry hyperplane. As a result, certain boundary conditions are satisfied automatically by the presence of a mirror image, greatly facilitating the solution of the original problem.
Method of image charges
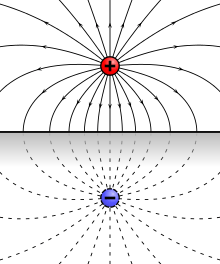
The method of image charges is used in electrostatics to simply calculate or visualize the distribution of the electric field of a charge in the vicinity of a conducting surface. It is based on the fact that the tangential component of the electrical field on the surface of a conductor is zero, and that an electric field E in some region is uniquely defined by its normal component over the surface that confines this region (the uniqueness theorem).[1]
Magnet-superconductor systems
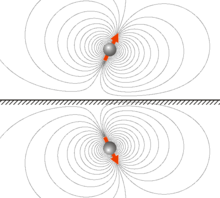
The method of images may also be used in magnetostatics for calculating the magnetic field of a magnet that is close to a superconducting surface. The superconductor in so-called Meissner state is an ideal diamagnet into which the magnetic field does not penetrate. Therefore, the normal component of the magnetic field on its surface should be zero. Then the image of the magnet should be mirrored. The force between the magnet and the superconducting surface is therefore repulsive.
Comparing to the case of the charge dipole above a flat conducting surface, the mirrored magnetization vector can be thought as due to an additional sign change of an axial vector.
In order to take into account the magnetic flux pinning phenomenon in type-II superconductors, the frozen mirror image method can be used.[2]
References
- ↑
- J. D. Jackson (1998). Classical Electrodynamics (3rd ed.). John Wiley & Sons. ISBN 978-0-471-30932-1.
- ↑ Kordyuk, A. A. (1998). "Magnetic levitation for hard superconductors" (PDF). Journal of Applied Physics. 83: 610–611. Bibcode:1998JAP....83..610K. doi:10.1063/1.366648.