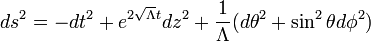Kantowski-Sachs metric

In general relativity the Kantowski-Sachs metric describes a homogeneous but anisotropic universe whose spatial section has the topology of 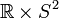\mathbb{R} \times S^{2}. The metric is:

ds^{2} = -dt^{2} + e^{2\sqrt{\Lambda}t} dz^{2} + \frac{1}{\Lambda}(d\theta^{2} + \sin^{2}\theta d\phi^{2})

The isometry group of this spacetime is 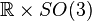\mathbb{R} \times SO(3). Remarkably, the isometry group does not act simply transitively on spacetime, nor does it possess a subgroup with simple transitive action.

See also


This article is issued from Wikipedia - version of the 6/22/2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.