Initial topology

In general topology and related areas of mathematics, the initial topology (or weak topology or limit topology or projective topology) on a set $X$ , with respect to a family of functions on $X$ , is the coarsest topology on X that makes those functions continuous.

The subspace topology and product topology constructions are both special cases of initial topologies. Indeed, the initial topology construction can be viewed as a generalization of these.

The dual construction is called the final topology.

Definition

Given a set X and an indexed family (Y_i)_i∈I of topological spaces with functions

f_{i}:X\to Y_{i}

the initial topology τ on $X$ is the coarsest topology on X such that each

f_{i}:(X,\tau )\to Y_{i}

is continuous.

Explicitly, the initial topology may be described as the topology generated by sets of the form $f_{i}^{{-1}}(U)$ , where $U$ is an open set in $Y_{i}$ . The sets $f_{i}^{{-1}}(U)$ are often called cylinder sets. If I contains exactly one element, all the open sets of $(X,\tau )$ are cylinder sets.

Examples

Several topological constructions can be regarded as special cases of the initial topology.

The subspace topology is the initial topology on the subspace with respect to the inclusion map.
The product topology is the initial topology with respect to the family of projection maps.
The inverse limit of any inverse system of spaces and continuous maps is the set-theoretic inverse limit together with the initial topology determined by the canonical morphisms.
The weak topology on a locally convex space is the initial topology with respect to the continuous linear forms of its dual space.
Given a family of topologies {τ_i} on a fixed set X the initial topology on X with respect to the functions id_i : X → (X, τ_i) is the supremum (or join) of the topologies {τ_i} in the lattice of topologies on X. That is, the initial topology τ is the topology generated by the union of the topologies {τ_i}.
A topological space is completely regular if and only if it has the initial topology with respect to its family of (bounded) real-valued continuous functions.
Every topological space X has the initial topology with respect to the family of continuous functions from X to the Sierpiński space.

Properties

Characteristic property

The initial topology on X can be characterized by the following characteristic property:
A function $g$ from some space $Z$ to $X$ is continuous if and only if $f_{i}\circ g$ is continuous for each i∈I.

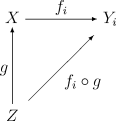
Note that, despite looking quite similar, this is not a universal property. A categorical description is given below.

Evaluation

By the universal property of the product topology, we know that any family of continuous maps f_i : X → Y_i determines a unique continuous map

f\colon X\to \prod _{i}Y_{i}\,.

This map is known as the evaluation map.

A family of maps {f_i: X → Y_i} is said to separate points in X if for all x ≠ y in X there exists some i such that f_i(x) ≠ f_i(y). Clearly, the family {f_i} separates points if and only if the associated evaluation map f is injective.

The evaluation map f will be a topological embedding if and only if X has the initial topology determined by the maps {f_i} and this family of maps separates points in X.

Separating points from closed sets

If a space X comes equipped with a topology, it is often useful to know whether or not the topology on X is the initial topology induced by some family of maps on X. This section gives a sufficient (but not necessary) condition.

A family of maps {f_i: X → Y_i} separates points from closed sets in X if for all closed sets A in X and all x not in A, there exists some i such that

f_{i}(x)\notin \operatorname {cl}(f_{i}(A))

where cl denoting the closure operator.

Theorem. A family of continuous maps {f_i: X → Y_i} separates points from closed sets if and only if the cylinder sets

f_{i}^{{-1}}(U)

, for U open in Y_i, form a base for the topology on X.

It follows that whenever {f_i} separates points from closed sets, the space X has the initial topology induced by the maps {f_i}. The converse fails, since generally the cylinder sets will only form a subbase (and not a base) for the initial topology.

If the space X is a T₀ space, then any collection of maps {f_i} which separate points from closed sets in X must also separate points. In this case, the evaluation map will be an embedding.

Categorical description

In the language of category theory, the initial topology construction can be described as follows. Let Y be the functor from a discrete category J to the category of topological spaces Top which selects the spaces Y_j for j in J. Let U be the usual forgetful functor from Top to Set. The maps {f_j} can then be thought of as a cone from X to UY. That is, (X, f) is an object of Cone(UY)—the category of cones to UY.

The characteristic property of the initial topology is equivalent to the statement that there exists a universal morphism from the forgetful functor

U′ : Cone(Y) → Cone(UY)

to the cone (X, f). By placing the initial topology on X we therefore obtain a functor

I : Cone(UY) → Cone(Y)

which is right adjoint to the forgetful functor U′. In fact, I is a right-inverse to U′ since U′I is the identity functor on Cone(UY).

References

Willard, Stephen (1970). General Topology. Reading, Massachusetts: Addison-Wesley. ISBN 0-486-43479-6.
"Initial topology". PlanetMath.
"Product topology and subspace topology". PlanetMath.

This article is issued from Wikipedia - version of the 12/19/2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.