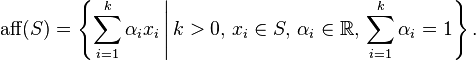Affine hull

In mathematics, the affine hull of a set S in Euclidean space Rⁿ is the smallest affine set containing S, or equivalently, the intersection of all affine sets containing S. Here, an affine set may be defined as the translation of a vector subspace.

The affine hull aff(S) of S is the set of all affine combinations of elements of S, that is,

\operatorname{aff} (S)=\left\{\sum_{i=1}^k \alpha_i x_i \, \Bigg | \, k>0, \, x_i\in S, \, \alpha_i\in \mathbb{R}, \, \sum_{i=1}^k \alpha_i=1 \right\}.

Examples

The affine hull of a singleton (a set made of one single point) is the singleton itself.
The affine hull of a set of two different points is the line through them.
The affine hull of a set of three points not on one line is the plane going through them.
The affine hull of a set of four points not in a plane in R³ is the entire space R³.

If instead of an affine combination one uses a convex combination, that is one requires in the formula above that all 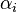$\alpha_i$ be non-negative, one obtains the convex hull of S, which cannot be larger than the affine hull of S as more restrictions are involved.
The notion of conical combination gives rise to the notion of the conical hull
If however one puts no restrictions at all on the numbers 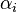$\alpha_i$ , instead of an affine combination one has a linear combination, and the resulting set is the linear span of S, which contains the affine hull of S.

This article is issued from Wikipedia - version of the 1/21/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.